Surge Pressure
Pipeline pressure surges are occurred when there is a sudden increase or decrease in pressure which is produced due to change in velocity of the moving fluid in a pipeline.
Due to instantaneous conversion of momentum to pressure when flowing liquid stopped quickly this sudden increase or surge of pressure is experienced. Pressure surge is popularly known as Water Hammer or Fluid Hammer or Hydraulic Surge.
The reasons for surge occurrences are as follows:
- Due to the events such as sudden shutting down of a pumping station or pumping unit.
- Unstable controls.
- Oscillation in tank levels.
- The sudden closure of a valve.
- Any other sudden blockage of the moving fluid.
These pressure surges may occur in all fluid pipeline systems and can result in pipeline fatigue and pipeline failure. The effects of surge may be catastrophic failure of the pipeline system and equipment or fatigue failure of the pipeline supports, instrumentation, equipment and compounds.
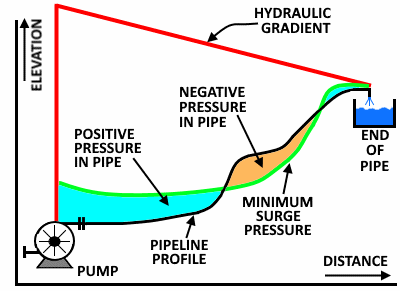
Fig 1: General schematic showing up-surge and down-surge pressure which can be generated in a pipeline.
Basic Definitions concerning Pressure Surge:
- Pressure Surge: It is basically a pressure wave caused due to a sudden change in flow velocity.
- Wave speed or acoustic velocity: The velocity at which pressure waves travel through the liquid/fluid.
- Joukowsky equation: Relationship relating head change to velocity change and acoustic velocity.
- Pipeline Period: Time required for a pressure wave to traverse the pipe/pipeline length and come back.
- Pressure Head: Pressure measured as the height of fluid (10 m head of water is roughly 1 atmosphere)
- Effective Valve closure Time: The period over which a Valve reduces the flow from 90% of its steady state to zero. In relation to Total Valve Closure Time, this is typically the last 15% opening for butterfly valves, 25% opening for ball valves and 30% opening for plug valves. This can be used as a rule of thumb at the initial assessment phases.
Methods of Analysis of Surge Pressure
- Method of Characteristics
- Wave Plan Method
Method of Characteristics
Joukowsky formula
The most important parameters to estimate the magnitude of transient pressures is:
- Acoustic wave speed, a
- Pipe/Pipeline period, T
- Joukowsky head, Δh
The acoustic wave speed formula depends on the fluid and the pipe characteristics expressed as:
- a = Velocity of the pressure wave
- K = Bulk modulus of the fluid
- ρ = Liquid density
- D = Internal diameter of the pipe
- E = Young’s modulus of the pipe material
- e = Wall thickness
- ϕ = restraint factor (usually taken as 1)

The time that a pressure wave takes to travel from its origin through the system and back to its source is defined as the pipe period. For a single pipeline with pipeline Length, L
this is provided as given below:
T = Critical period
L = Length of the pipe
a = Velocity of the pressure wave
Events that take place in less than T are called ‘fast’ events and these are likely to cause pressure surge issues
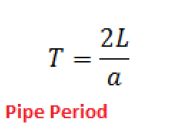

As per the Joukowsky formula, the pressure head change (Δh) due to an instantaneous velocity change (ΔV) is expressed as shown above. Here,
Δh = head rise
ΔV = change in velocity
a = wave speed
g = acceleration due to gravity
This is a very useful guide that explains the likely severity of a pressure surge event but is not a replacement for a proper surge analysis!
Limitations of Joukowsky formula
- Joukowsky formula is applicable to a limited set of fluid systems. It’s application should be limited to situations matching the following criteria:
- Simple ‘linear’ piping systems i.e., there are no branches by which pressure
- waves can be reflected back and cause constructive interference in the main line.
- Valve closure time is significantly shorter than the pressure wave communication time.
- System frictional losses are similar to that of a water transport system.
- Joukowsky equation does not consider column separation in its analysis of fluid hammer.
- Column separation can often result in surge pressures exceeding those predicted by the Joukowsky equation and therefore the Joukowsky equation should not be applied when analyzing a system in which the pipeline pressure can rapidly drop below the fluid vapor pressure.


